Using the Pythagorean Expectation to Evaluate the First Round of the NBA Playoffs
So Jimmy Butler might be Michael Jordan’s long-lost son after all. The first round of the playoffs has concluded, and seemingly out of nowhere, Jimmy Butler’s Miami Heat caught fire (no pun intended), ending the first-seed Milwaukee Bucks’ season. Despite having the worst 3-point percentage during the regular season, the Heat shot a staggering 60%, 48.5%, 40.6%, and 37.% percent in their four wins to defeat the Bucks in five.
Perhaps Butler and the Heat just happened to get the hot hand at the right time. Perhaps. Or maybe Milwaukee simply underperformed. It’s important to note that Giannis missed the first two games of the series. Perhaps the Bucks were never supposed to be the favorite, despite being seeded 7 spots ahead of the Heat.
Interested, I wanted to see if perhaps Miami was a better team than we had thought, or if Milwaukee was perhaps undeserving of their first seed.
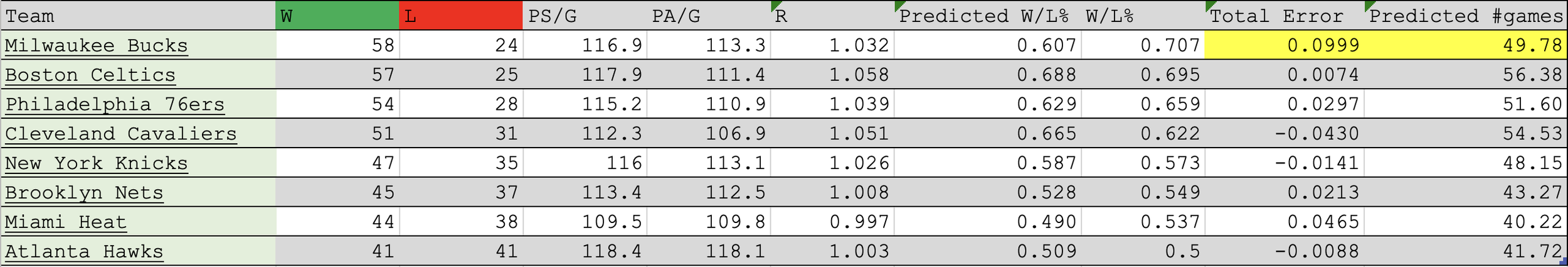
Over the course of the regular season, Milwaukee averaged 116.9 points scored per game and gave up on average 113.3 points scored per game. Miami on average scored 109.5 points per game, giving up 109.8 points per game. Whereas the Heat tend to give up more points than their opponents, Milwaukee comfortably outscores their opponents on a night-to-night basis. It makes sense that the Bucks stood atop the Eastern Conference with a record of 58 wins and 24 losses, standing atop the Eastern Conference. On the other hand, Miami finished with 44 wins and 38 losses, just making the cut for the playoffs.
But certainly, as you could imagine, this isn’t the full picture. See, Bill James, the biggest proponent of baseball mathematics, created a “Pythagorean Expectation” model, which argues that in baseball, a team’s winning percentage is related to the square of the runs scored by the team, divided by the sum of the runs scored squared and runs conceded squared. Darryl Morey, general manager of the 76ers and godfather of today’s annoyingly efficient high-volume 3-point and layup revolution, adopted this idea to basketball. But, instead of squaring the runs scored and runs conceded, he changed it for an exponent (or e value) that would minimize the root mean square error to better fit the basketball data. This is what he came up with:
This formula is another way to interpret a team’s win percentage, using the overall points scored and points allowed through the season, instead of just the final result of the games. We can use this data to create predicted winning percentages for each team, compare the predicted winning percentages to the actual winning percentage during the regular season, and see if this gives any indication of how a team performed in the playoffs. Here’s the Eastern Conference:
Key: PS/G: points scored per game; PA/G; points allowed per game; Predicted W/L%: the win percentage obtained from the Pythagorean expectation; W/L%: the actual team’s win percentage ***other variables are explained in the following
R is the PS/G divided by the PA/G, or PS/PA. If you took Morrey’s Win% formula and divided both the numerator by runs allowed^13.91, then what you would get is the equation of: R^13.91/(R^13.91+1), which is the formula to find the predicted win percentage. Using this formula we found that the predicted win percentage of the Bucks is less than their actual win percentage by 0.0999% (Total Error variable). Essentially, what we have here is that the Bucks’ actual win percentage is essentially 10 percent greater than what we would have predicted based on just their points scored and points allowed. Following this model, we would have predicted the Bucks to win 49.78, or 50 games, but have won 8 more games in reality. In some sense, you could interpret it as the following: Based on the ability to score points and prevent opponents from scoring points, we would expect the Milwaukee Bucks to win 50 games. According to the Pythagorean expectation, in this regular season, the Bucks have seemed to out-win their ability. Interesting.
If we re-organize the standings, the Heat would actually be dead last (note that despite winning more games than the Hawks, the Heat were the 8th seed in the 1st round due to the play-in tournament) but the Bucks would only rank fourth, behind the Celtics, Cavs, and 76ers. While taking nothing away from Butler and co.’s performance, we can also make the claim that the Bucks were over-ranked in the first place. Rather than looking at this first-round miracle as an 8-seed defeating a 1-seed in five games, look at it as an 8-seed routing a 4th-seed. Again, taking nothing away from the Heat’s unbelievable performance.
It’s important to note that this sort of work is not new at all. Though I’m not sure if he invented this analysis, author and data scientist Wayne L. Winston, in his book Mathletics, used data from the 2006-2007 season to show that “the Miami Heat and Dallas Mavericks [ranked 4th and 1st in the Eastern and Western Conference, respectively] both won about 8% more games than expected during the regular season. Therefore, [one] would have predicted Miami and Dallas to perform worse during the playoffs than their actual win-loss record indicated.” Sure enough, reigning MVP Dirk Nowitzki and the first-seeded Dallas Mavericks were bumped in the first round to the ‘We Believe’ Warriors, and the 06-07 Miami Heat were swept in four games by the Chicago Bulls. This phenomenon also seems to perform the other way, as the Chicago Bulls, the fifth seed in the Eastern Conference, “won around 8% fewer games than the Pythagorean Theorem [predicted], indicating that these teams would perform better than expected in the playoffs.
If we look at the Western Conference predicted win percentages, we can see traces of this “underperformance” at play. Take for example the Phoenix Suns and the Los Angeles Clippers, the 4th and 5th seeds. While the Suns were already seeded ahead of the Clippers (by one game) perhaps the gap should’ve been larger than we expected.
As you can tell, The Phoenix Suns performed worse than their record would indicate in the regular season, while the Clippers essentially overperformed by 2.17%. If we reranked the Western Conference using the Pythagorean Expectation predicted win percentage, we would see the Suns ranked 3rd and the Clippers ranked 7th. Perhaps we should’ve known all along that the Suns would take home the series in 5.
Furthermore, while not the most eye-catching data, it’s also interesting to see the Warriors, the 7th seed, who underperformed during the regular season by 1.6% defeat the Kings, who overperformed by 1%, in a very competitive 7-game series. Adjusted to the Pythagorean Expectation, we would have seen the Warriors rank fifth in the Western Conference.
Of course, this is merely anecdotal data, and let’s not jump to conclusions that the Pythagorean Expectation adjusted rankings are the end-all-be-all to the playoffs—even parts of this data set prove it isn’t. But it wouldn’t be a stretch to say that this data tells an interesting story—one to look out for as the rest of the playoffs continue.
Sources:
Mathletics by Wayne L. Winston
NBA.com
https://en.wikipedia.org/wiki/Pythagorean_expectation